I recently was a guest speaker for a new teacher college course. I shared some Tips and Tricks I've learned in my 10 years as an ESL teacher. Screen shots of the slides are below, and you can see the compete show here: Tips and Tricks from an ESL Teacher
Mrs. Waugh Barrios
Education is the most powerful weapon for changing the world.

Welcome to Ms. Waugh's Class Blog
Welcome students, parents, and colleagues. Thank you for visiting my blog! Here are many resources for teaching ESL students.
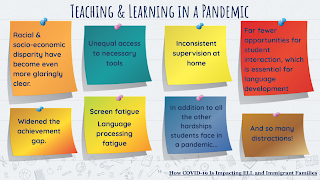
Friday, June 4, 2021
Wednesday, October 24, 2018
Vocabulary Strategies 101
To see the slideshow presentation, click here: Vocabulary Strategies 101
Last week, I presented at a half-day PD at Cameron Middle School this presentation on easily integratable Vocabulary Strategies in the SEI classroom. Here is the presentation for your reference.
Last week, I presented at a half-day PD at Cameron Middle School this presentation on easily integratable Vocabulary Strategies in the SEI classroom. Here is the presentation for your reference.
Monday, October 22, 2018
Weekly ELD Strategy #6
I was talking with a 6th grade science teacher, who said her students were really struggling to comprehend the vocabulary of a particular unit. She said they were learning about mollusks, and I said, "You know mollusk is a Spanish and Portuguese cognate, right?"
A what?
A cognate is a word that shares a similar meaning, spelling, and pronunciation in two or more languages. Since Spanish and Portuguese are both Romance languages, they have many, many cognates, and many English words are cognates with Spanish and Portuguese. In fact, 30-40% of all words in English have a related word in Spanish, and this cognate awareness can really help ELs understand their second language.
Strategy 6: Teaching Cognates
Unless you are fluent in Spanish or Portuguese, how can you teach cognates to your students? Colorin Colorado has a few good ideas.
- Create a class chart or word wall or digital cognate thesaurus as a reference and repository for any cognates you or your students find during a lesson or unit
- When reading aloud, ask ELs to point out when they think they hear a cognate. Stop reading and discuss that cognate, and then add those words to your class list
- When students are reading texts in their content classes, encourage them to write on a sticky note any cognate they see. These words could also be added to the chart.
- Reinforce cognates you have already discussed by having ELs do a word sort.
- Have students circle the differences between the cognates by identifying which letters are different. This also helps students bridge new vocabulary in one or both languages to their background knowledge in L1
Note: Beware of False Cognates!
Not all words that sound similar in Spanish/Portuguese and English actually have the same meaning. (Example embarazada does not mean embarrassed, it means pregnant!) This, however, is also a teachable moment. (See 20 False Cognates that Can Get You in Trouble)
Click here for a list of 1001 English-Spanish Cognates
**As always, all of the ELD Weekly Strategies can be found here, for your reference. If you have any questions about this ELD strategy or any other strategy, please let me know.
Weekly ELD Strategy #5
This week’s ELD strategy is brought to you by the Blue Team at Fuller Middle School.
Strategy 5: Scaffold Participation with Sentence starters for Clarification
In a meeting last week, they showed me this desktop anchor chart they use to help scaffold student participation and self-advocacy, and I asked if I could share it as my weekly strategy.
In the early days, months, and years of being integrated into US schools or mainstream English classes, ELs can have a very high affect filter, which keeps them from participating in class, and most importantly, asking for help when they don’t understand. In order to combat and support this silent period, the Blue Team developed these sentence starters to help students be able to have the vocabulary necessary to ask for clarification during a lesson.
This is little chart students can keep on their desk and reference it whenever they need it. The Blue Team provided a copy for every desk, and made it even more durable by slipping the sheet into a recycled name tag from the Keefe Field Trips last year.
I encourage you and your teams to think about what academic language is necessary in your classes, and how you can support this production from your ELs.
Thank you Blue Team, for sharing this great strategy!
**As always, all of the ELD Weekly Strategies can be found here, for your reference. If you have any questions about this ELD strategy or any other strategy, please let me know.
Weekly ELD Strategy #4
Week 4: October 5, 2018
ELD Strategy 4: Interactive Dictionary
Vocabulary acquisition is an area where our ELs can really struggle.
Here is a chart of Vocabulary Usage expectations by WIDA Level,
which is adapted from the WIDA Speak and Writing rubrics,
and shows what ELs can produce orally and in writing at each WIDA level.
But how do we help them expand these vocabularies?
Here is a chart of Vocabulary Usage expectations by WIDA Level,
which is adapted from the WIDA Speak and Writing rubrics,
and shows what ELs can produce orally and in writing at each WIDA level.
But how do we help them expand these vocabularies?
ELs of all levels are constantly encountering new vocabulary.
One way to help them organize this new information is to develop an Interactive Dictionary.
This could be something students do individually, or by class, or team, or grade.
And now that almost every classroom has one-to-one chromebooks,
creating a digital interactive dictionary could be a resource easily shared among all of the ELs.
One way to help them organize this new information is to develop an Interactive Dictionary.
This could be something students do individually, or by class, or team, or grade.
And now that almost every classroom has one-to-one chromebooks,
creating a digital interactive dictionary could be a resource easily shared among all of the ELs.
Often times we as teachers define many new words during the course of one lesson.
I was in an ELA class this week and the teacher had to define five words for the ELs on just one page of the Outsiders!
However, research shows students need multiple exposures to new words in order to move them into their long-term memories.
Keeping an Interactive Dictionary can help reinforce our students’ burgeoning vocabularies,
and be a support for them to look back on as we ask them to analyze and synthesize previously read text or content.
Here is an example below.
I was in an ELA class this week and the teacher had to define five words for the ELs on just one page of the Outsiders!
However, research shows students need multiple exposures to new words in order to move them into their long-term memories.
Keeping an Interactive Dictionary can help reinforce our students’ burgeoning vocabularies,
and be a support for them to look back on as we ask them to analyze and synthesize previously read text or content.
Here is an example below.
word
|
reference
|
meaning
|
drugstore
|
The Outsiders, Ch 2 p.18
|
Like a pharmacy, where they sell medicine
|
Kools
|
The Outsiders, Ch 2 p.18
|
A brand of cigarettes
|
drive-ins
|
The Outsiders, Ch 2 p.18
|
A place where you watch a movie in your car
|
fuzz
|
The Outsiders, Ch 2 p.18
|
The police
|
Concession stand
|
The Outsiders, Ch 2 p.18
|
Where you can buy snacks
|
The key is to record the student-friendly definitions we share with our ELs in the moment.
Optimally, this chart would be projected during the lesson,
and the teacher or a selected student could record the words and their meanings in real-time.
(Then even to differentiate it further, teachers could assign students at the lower WIDA levels to find an image that defines each word.)
Optimally, this chart would be projected during the lesson,
and the teacher or a selected student could record the words and their meanings in real-time.
(Then even to differentiate it further, teachers could assign students at the lower WIDA levels to find an image that defines each word.)
**As always, all of the ELD Weekly Strategies can be found here, for your reference. If you have any questions about this ELD strategy or any other strategy, please let me know.
Weekly ELD Strategy #3
Week 3: September 26, 2018
ELD Strategy 3: How to Write Language Objectives When You are Stuck
When writing Language Objectives, there is a lot to think about, including the language skills necessary for students to accomplish the lesson, grammar or language structures common in the content area, the tasks the students need to complete and the language embedded in those assignments, and any language learning strategies necessary to the topic of the lesson. All of these considerations can be totally overwhelming! So when you are struggling to write a language objective, this strategy can present a great way to start.
First, determine which macro function of language you want the students to focus on for the lesson: Recount, Explain, Argue, Discuss. (Just remember the acronym READ)
Then look at the Can Do Descriptors Key Uses Edition. This is a document from WIDA that provides examples of what ELs can do at each WIDA level. It is organized by the key uses (Recount p.4-5, Explain p.6-7, Argue p.8-9 and Discuss p.10) and by the language domain (reading, writing, speaking, listening.)
The columns are arranged by "ELP Level," which stands for "English Language Proficiency Level." If you have a class with a majority of Wida 3's, you can look at ELP Level 3 or ELP Level 4, and use those can do descriptors to phrase your language objectives.
Example,
- Students will recount by reading the text and identifying topic sentences, main ideas, and details in paragraphs
- Students will explain by stating why events occur, phenomena exist, or some things happen
- Students will argue by crafting persuasive pieces (e.g., editorials) with a series of substantiated content-related claims
- Students will discuss by answering Wh- questions in conversations
(Note: These can-do descriptors are intentionally written broadly, so they can be rephrased for your specific content)
It is also important to include a tag of how students will demonstrate that they have met the objective, like "orally" or "in writing" or "by completing a graphic organizer."
*Please also reference Fuller's guidelines for Writing Effective Learning Objectives
*To learn more, check out this article: Language Objectives: The Key to Effective Content Area Instruction for English Learners.
*The DESE provides a really helpful framework for writing focus language goals called Collaboration Tool, and while the purpose of this document is designed for writing unit-level goals, it can easily be adopted for writing language objectives.
**As always, all of the ELD Weekly Strategies can be found here, for your reference. If you have any questions about this ELD strategy or any other strategy, please let me know.
Weekly ELD Strategy #2
Week 2: September 18, 2018
Strategy 2: X2 Tutorial for EL Information
When you are looking at your class list on X2, a large globe next to the student name indicates EL, and a small globe means former EL (also called FEL.) In this way, you can quickly see how many ELs and FELs you have in each section.
To see when a student entered and/or exited the ESL program (or SPED program), look under the "Services" tab, under the "details" page.
All of a student's ACCESS scores are listed under the "Assessments" tab.
If you click on the date of the assessment (example: 1/22/2018), you can see the individual student score report by domain. This is also how we identify a student's WIDA level. This student's overall proficiency is 1.8, so she would be a WIDA 2.
**To learn more about what a WIDA 2 student can do, please reference the WIDA CAN DO Descriptors for Reading, Writing, Speaking and Listening.**
**As always, all of the ELD Weekly Strategies can be found here, for your reference. If you have any questions about this ELD strategy or any other strategy, please let me know.
Subscribe to:
Posts (Atom)